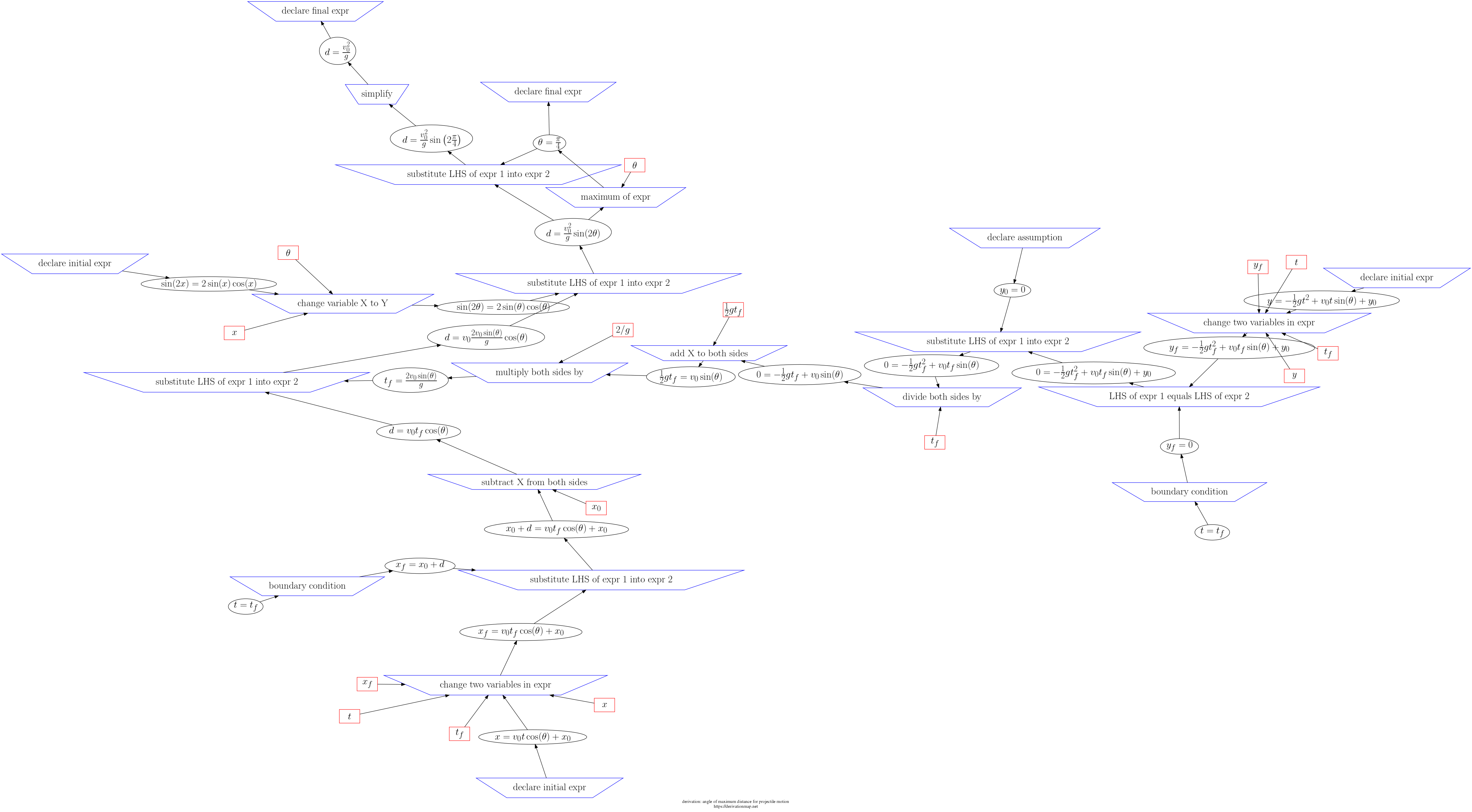
This page contains three views of the steps in the derivation: d3js, graphviz PNG, and a table.
Notes for this derivation:
| Index | Inference Rule | Input latex | Feeds latex | Output latex | step validity | dimension check | unit check | notes |
|---|---|---|---|---|---|---|---|---|
| 7 | divide both sides by |
|
|
|
valid |
1087417579:
2086924031: |
1087417579:
2086924031: |
|
| 4 | LHS of expr 1 equals LHS of expr 2 |
|
|
|
input diff is 0 diff is -pdg1469 + pdg1649*pdg2467**2/2 - pdg2467*pdg5153*sin(pdg1575) diff is pdg1469 - pdg1649*pdg2467**2/2 + pdg2467*pdg5153*sin(pdg1575) |
5379546684:
9112191201: 8198310977: |
5379546684:
9112191201: 8198310977: |
|
| 22 | declare final expr |
|
|
|
no validation is available for declarations |
5353282496:
|
5353282496:
|
|
| 16 | declare initial expr |
|
|
|
no validation is available for declarations |
2405307372:
|
2405307372:
|
|
| 10 | declare initial expr |
|
|
|
no validation is available for declarations |
5438722682:
|
5438722682:
|
|
| 21 | simplify |
|
|
|
LHS diff is 0 RHS diff is pdg5153**2*(sin(pdg3141/2) - 1)/pdg1649 |
3607070319:
5353282496: |
3607070319:
5353282496: |
|
| 12 | boundary condition |
|
|
|
no validation is available for assumptions |
4370074654:
2378095808: |
4370074654:
2378095808: |
|
| 13 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
2378095808:
3485125659: 4268085801: |
2378095808:
3485125659: 4268085801: |
|
| 14 | subtract X from both sides |
|
|
|
valid |
4268085801:
7233558441: |
4268085801:
7233558441: |
|
| 11 | change two variables in expr |
|
|
|
valid |
5438722682:
3485125659: |
5438722682:
3485125659: |
|
| 2 | change two variables in expr |
|
|
|
valid |
9862900242:
5379546684: |
9862900242:
5379546684: |
|
| 6 | substitute LHS of expr 1 into expr 2 |
|
|
|
LHS diff is pdg1469 RHS diff is pdg1469 |
8198310977:
1650441634: 1087417579: |
8198310977:
1650441634: 1087417579: |
|
| 18 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
2519058903:
2297105551: 8922441655: error for dim with 8922441655 |
2519058903:
2297105551: 8922441655: N/A |
|
| 3 | boundary condition |
|
|
|
no validation is available for assumptions |
5373931751:
9112191201: |
5373931751:
9112191201: |
y(t_f) = y_f = 0 |
| 23 | declare final expr |
|
|
|
no validation is available for declarations |
1541916015:
dimensions are consistent |
1541916015:
N/A |
|
| 9 | multiply both sides by |
|
|
|
valid |
1191796961:
4778077984: |
1191796961:
4778077984: |
|
| 15 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
4778077984:
7233558441: 2297105551: |
4778077984:
7233558441: 2297105551: |
|
| 1 | declare initial expr |
|
|
|
no validation is available for declarations |
9862900242:
|
9862900242:
|
|
| 8 | add X to both sides |
|
|
|
valid |
2086924031:
1191796961: |
2086924031:
1191796961: |
|
| 20 | substitute LHS of expr 1 into expr 2 |
|
|
|
valid |
1541916015:
dimensions are consistent 8922441655: error for dim with 8922441655 3607070319: |
1541916015:
N/A 8922441655: N/A 3607070319: |
|
| 5 | declare assumption |
|
|
|
no validation is available for declarations |
1650441634:
|
1650441634:
|
|
| 17 | change variable X to Y |
|
|
|
LHS diff is sin(2*pdg1464) - sin(2*pdg1575) RHS diff is sin(2*pdg1464) - sin(2*pdg1575) |
2405307372:
2519058903: |
2405307372:
2519058903: |
|
| 19 | maximum of expr |
|
|
|
no check performed |
8922441655:
error for dim with 8922441655 1541916015: dimensions are consistent |
8922441655:
N/A 1541916015: N/A |